55 7.7 Power
Summary
- Calculate power by calculating changes in energy over time.
- Examine power consumption and calculations of the cost of energy consumed.
What is Power?
Power—the word conjures up many images: a professional football player muscling aside his opponent or a dragster roaring away from the starting line.
These images of power have in common the rapid performance of work, consistent with the scientific definition of power (P) as the rate at which work is done.
POWER
Power is the rate at which work is done.
The SI unit for power is the watt (W), where 1 watt equals 1 joule/second (1 W = 1 J/s).
Because work is energy transfer, power is also the rate at which energy is expended. A 60-W light bulb, for example, expends 60 J of energy per second. Great power means a large amount of work or energy developed in a short time. For example, when a powerful car accelerates rapidly, it does a large amount of work and consumes a large amount of fuel in a short time.
Calculating Power from Energy
Example 1: Calculating the Power to Climb Stairs
What is the power output for a 60.0-kg woman who runs up a 3.00 m high flight of stairs in 3.50 s, starting from rest but having a final speed of 2.00 m/s? (See Figure 2.)
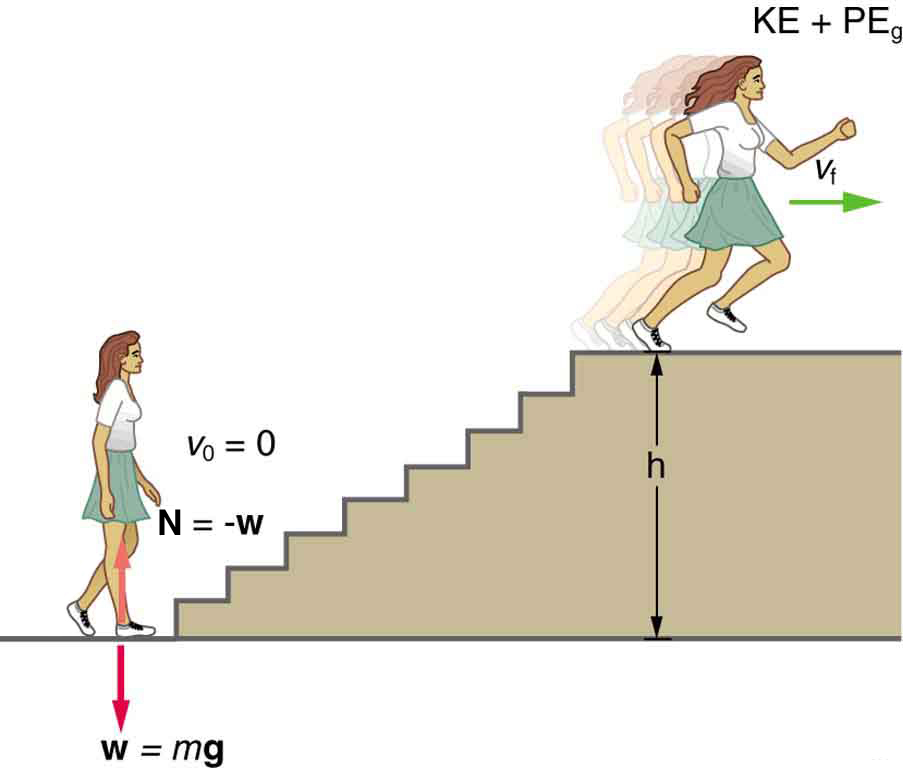
Strategy and Concept
The work going into mechanical energy is W = KE + PE. At the bottom of the stairs, we take both KE and PEgas initially zero; thus, [latex]\boldsymbol{W=\textbf{KE}_{\textbf{f}}+\textbf{PE}_{\textbf{g}}=\frac{1}{2}{mv_{\textbf{f}}}^2+mgh},[/latex] where h is the vertical height of the stairs. Because all terms are given, we can calculate W and then divide it by time to get power.
Solution
Substituting the expression for W into the definition of power given in the previous equation, P = W/t yields
Entering known values yields
Discussion
The woman does 1764 J of work to move up the stairs compared with only 120 J to increase her kinetic energy; thus, most of her power output is required for climbing rather than accelerating.
It is impressive that this woman’s useful power output is slightly less than 1 horsepower (1 hp = 746 W)! People can generate more than a horsepower with their leg muscles for short periods of time by rapidly converting available blood sugar and oxygen into work output. (A horse can put out 1 hp for hours on end.) Once oxygen is depleted, power output decreases and the person begins to breathe rapidly to obtain oxygen to metabolize more food—this is known as the aerobic stage of exercise. If the woman climbed the stairs slowly, then her power output would be much less, although the amount of work done would be the same.
MAKING CONNECTIONS: TAKE-HOME INVESTIGATION—MEASURE YOUR POWER RATING
Determine your own power rating by measuring the time it takes you to climb a flight of stairs. We will ignore the gain in kinetic energy, as the above example showed that it was a small portion of the energy gain. Don’t expect that your output will be more than about 0.5 hp.
Section Summary
- Power is the rate at which work is done, or in equation form, for the average power P for work W done over a time t, P = W/t.
- The SI unit for power is the watt (W), where 1 W = 1 J/s.
Problems & Exercises
1: A person in good physical condition can put out 100 W of useful power for several hours at a stretch, perhaps by pedalling a mechanism that drives an electric generator. Neglecting any problems of generator efficiency and practical considerations such as resting time: (a) How many people would it take to run a 4.00-kW electric clothes dryer? (b) How many people would it take to replace a large electric power plant that generates 800 MW?
2: (a) What is the average useful power output of a person who does 6.00 × 106 J of useful work in 8.00 h? (b) Working at this rate, how long will it take this person to lift 2000 kg of bricks 1.50 m to a platform? (Work done to lift his body can be omitted because it is not considered useful output here.)
Glossary
- power
- the rate at which work is done
- watt
- (W) SI unit of power, with 1 W = 1 J/s
- kilowatt-hour
- (kW • h) unit used primarily for electrical energy provided by electric utility companies
Solutions
Problems & Exercises
1: (a) 40 (b) 8 million
2: (a) 208 W (b) 141 s

