32 5.2 Angular Velocity
Angular Velocity
How fast is an object rotating? We define angular velocity ω as the rate of change of angular displacement. In symbols, this is
where an angular rotation Δθ takes place in a time Δt. The greater the rotation angle in a given amount of time, the greater the angular velocity. The units for angular velocity are degrees per second (º/s), radians per second (rad/s) or revolutions per minute (rpm) where applicable.
Angular velocity is a vector quantity. Angular velocity has only two directions with respect to the axis of rotation—it is either clockwise or counterclockwise.
Angular velocity is used in two ways in biomechanics. We are either interests in the average angular velocity or the instantaneous angular velocity. Average angular velocity tells us how long it takes for something to rotate through a certain angular displacement. Instantaneous angular velocity tells us how fast something is spinning at a specific instant in time. The average angular velocity of a tennis player’s swing might determine whether or not she contacts the ball but it is the racket’s instantaneous velocity at ball contact that determines how fast and how far the ball will go. In sports where whole body rotations are important (diving, gymnastics), angular velocity is an important determinant of whether or not the athlete will complete a certain number of twists or somersaults before landing.
Angular and Linear Velocity
In several sports, especially in those where an implement is used as an extension of the athlete’s limbs (golf, tennis, lacrosse..), the relationship between angular and linear velocity become important. The advantage of using implements is that they amplify the movement (displacement) of our limbs. Take a tennis ball and throw it as far as you can. Now hit that same ball with a tennis racket. Which goes the furthest? The racket enable faster linear velocities because they increase the distance from the point of contact (your hand vs the tennis racket) from the axis of rotation (shoulder joint). The relationship between linear variables, angular variables and the radius discussed in the previous section is important here as well.
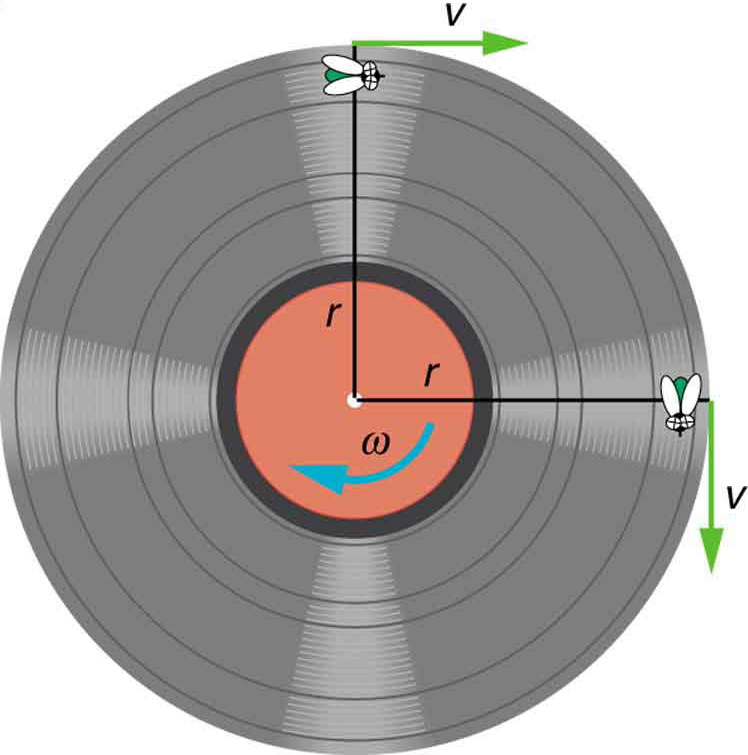
The first relationship in v = rω or ω = v / r states that the linear velocity v is proportional to the distance from the centre of rotation, thus, it is largest for the point furthest away from the point of rotation. The second relationship states that the faster an object rotates (ω), the faster the linear velocity of a point on the object (v). Note that in order to use this equation, angular velocity must be expressed in rads/s.
Both[latex]\boldsymbol{\omega}[/latex]and[latex]\boldsymbol{v}[/latex]have directions (hence they are angular and linear velocities, respectively). Angular velocity has only two directions with respect to the axis of rotation—it is either clockwise or counterclockwise. Linear velocity is tangent to the path.
Applying the relationship between linear and angular velocity to sport
Think of your favourite sports and the equipment required to play. If the sport you are thinking about includes the use of sticks, clubs or rackets you are likely already familiar with the relationship between linear and angular velocities. The linear velocity of a point farther from the axis of rotation is faster if the angular velocity is the same. This linear velocity gets passed along to the ball (or projectile) through the conservation of momentum which will be discussed later. In golf for example, we have two types of clubs: the woods and irons. The woods are the longest clubs and are used to impart faster velocity to the ball as the player drives the ball as far as possible. The irons are shorter clubs, used for closer shots.
You may not always be switching between a long and a shorter implement to affect the linear velocity of the projectile but you can also change the axis of rotation to shorten the radius. Let’s say a swing typically originates in the shoulder. By rotating about the wrist you are shortening the radius.
Perhaps you could also move your grip on the apparatus to shorten or lengthen the radius. This is seen commonly in baseball as the players choke up on the bat.
PHET EXPLORATIONS: LADYBUG REVOLUTION

Join the ladybug in an exploration of rotational motion. Rotate the merry-go-round to change its angle, or choose a constant angular velocity or angular acceleration. Explore how circular motion relates to the bug’s x,y position, velocity, and acceleration using vectors or graphs.
- Angular velocity ω is the rate of change of an angle,
[latex]\boldsymbol{\omega\:=}\boldsymbol{\frac{\Delta\theta}{\Delta{t}}},[/latex]
where a rotation Δθ takes place in a time Δt. The units of angular velocity are radians per second (rad/s). Linear velocity v and angular velocity ω are related by
[latex]\boldsymbol{v=r\omega\textbf{ or }\omega\:=}\boldsymbol{\frac{v}{r}}.[/latex] - The relationship between linear and angular velocities is expressed in the following equation: v = rω (angular velocity must be expressed in rads/s)
Problems & Exercises
1: A baseball pitcher brings his arm forward during a pitch, rotating the forearm about the elbow. If the velocity of the ball in the pitcher’s hand is 35.0 m/s and the ball is 0.300 m from the elbow joint, what is the angular velocity of the forearm?
2: In lacrosse, a ball is thrown from a net on the end of a stick by rotating the stick and forearm about the elbow. If the angular velocity of the ball about the elbow joint is 30.0 rad/s and the ball is 1.30 m from the elbow joint, what is the velocity of the ball?
3: Integrated Concepts
When kicking a football, the kicker rotates his leg about the hip joint.
(a) If the velocity of the tip of the kicker’s shoe is 35.0 m/s and the hip joint is 1.05 m from the tip of the shoe, what is the shoe tip’s angular velocity?
(b) The shoe is in contact with the initially stationary 0.500 kg football for 20.0 ms. What average force is exerted on the football to give it a velocity of 20.0 m/s?
(c) Find the maximum range of the football, neglecting air resistance. Remember there is an equation for maximum range. It happens when the launch angle is 45 degrees above horizontal.
- angular velocity
- ω, the rate of change of the angle with which an object moves on a circular path
Solutions
Problems & Exercises
1: 117 rad/second
3: (a) 33.3 rad/s (b) 500 N (c) 40.8 m

