"

F

Audio

Listen to an audio version of this page (2 min 55 sec):

 

Factor

piskic akitāsona

Definition: Factors are numbers or expressions we can multiply together to get another number.

Examples

  • 4 × 5 = 20; then 4 and 5 are factors of 20.
  • 2 × 3 × 7 = 42; then 2, 3 and 7 are factors of 42.
  • 3 × n = 20; then 3 and n are factors of 20.

Factoring

pa piskicipita

Definition: It is the process of finding the factors for a number or expression.

Example

Find the factors for 5x-20, 24, and 36.

  • [latex]5x-20=5(x-4)[/latex]. Therefore, the factors of 5x-20 are 5 and x-4.
  • [latex]24=4×6[/latex]. Therefore, the factors of 24 are 4 and 6.
  • [latex]36=2×2×3×3[/latex]. Therefore, the factors of 36 are 2, 2, 3, and 3.

Fifteen

neyânanosâp

Definition: It is a counting number that is one more than fourteen. It is written as 15.

Fifteen blackberries are laid on a surface. There are five in a row and three in a column.
Figure 6.1 Fifteen blackberries (illustration: Larissa Kitchemonia).

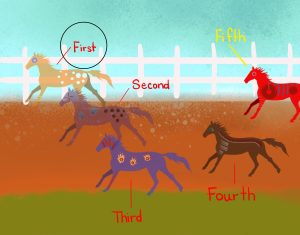
Fifth

mwecinîyânan

Definition: It is the number five in a sequence. It is also written as 5th.

Five horses are racing. The fastest one is labelled as first. The ones follow are labelled as second, third, fourth, and fifth. The horse running in number five in the race is labelled as fifth.
Figure 6.2 Visual representation of ordinal numbers in horse racing (illustration: Larissa Kitchemonia).

Fifty

nîyânanomitanaw

Definition: It is a counting number equivalent to five sets of tens. It is written as 50.

Fifty hazelnuts are laid on a surface. There are 10 in a row and five in a column.
Figure 6.3 Fifty hazelnuts (illustration: Larissa Kitchemonia).

First

mwecipeyakwâw

Definition: It is before anything else, constituting number one in a sequence.

Five horses are racing. The fastest one is labelled as first. The ones follow are labelled as second, third, fourth, and fifth. The fastest running horse is labelled as first.
Figure 6.4 Visual representation of ordinal numbers in horse racing (illustration: Larissa Kitchemonia).

Five

nîyânan

Definition: It is a counting number that is one more than four. It is written as 5.

Five blue flowers are laid on the surface.
Figure 6.5 Five blue flowers (illustration: Larissa Kitchemonia).

Form

kayisenakwahk

Definition: The manner or style of arranging and coordinating parts [6].

Examples

  • standard form: [latex]3x+2y=7[/latex]
  • exponential form: [latex]3×3×3×3×3=3^5[/latex]
  • expanded form: [latex]537=5×100+3×10+7×1[/latex]

Forty

nêwomitanaw

Definition: It is a counting number equivalent to four sets of tens. It is written as 40.

Forty hazelnuts are laid on the surface. There are ten in a row and four in a column.
Figure 6.6 Forty hazelnuts (illustration: Larissa Kitchemonia).

Four

nêwo

Definition: It is a counting number that is one more than three.

A hand with four fingers up.
Figure 6.7 A hand with four fingers up (illustration: Larissa Kitchemonia).

Fourth

mwecinewiyihk

Definition: It is the number four in a sequence.

Fourteen

nêwosâp

Definition: It is a counting number that is one more than thirteen. It is written as 14.

Fourteen blackberries.
Figure 6.8 Fourteen blackberries (illustration: Larissa Kitchemonia).

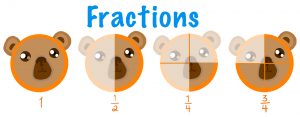
Fraction

pahki akihtāson

Definition: A fraction represents a part of a whole object. It is written with a number on the top of a line, called a numerator and another number on the bottom, called a denominator.

Examples of Fractions

  • [latex]\frac{3}{4}[/latex]
  • [latex]\frac{x}{2y}[/latex]
  • [latex]\frac{2x-1}{3x^2+7}[/latex]

 

There are four examples of fractions. They are 1, 1/2, 1/4, and 3/4.
Figure 6.9 Examples of fractions (illustration: Larissa Kitchemonia).

License

Icon for the Creative Commons Attribution 4.0 International License

Cree Dictionary of Mathematical Terms with Visual Examples and Sound Copyright © 2024 by Arzu Sardarli is licensed under a Creative Commons Attribution 4.0 International License, except where otherwise noted.

Share This Book